Special Parallelograms

A parallelogram may be equiangular (rectangle), equilateral (rhombus) or both equiangular and equilateral. An example of a special parallelogram that is both equiangular and equilateral is our friend the square.

Rectangle

A rectangle is a parallelogram with 4 right angles. A rectangle has the following rules:
(1) All the rules of a parallelogram.
(2) Four right angles. Remember that a right angle measures 90 degrees.
(3) Diagonals which are congruent (they have the same length).
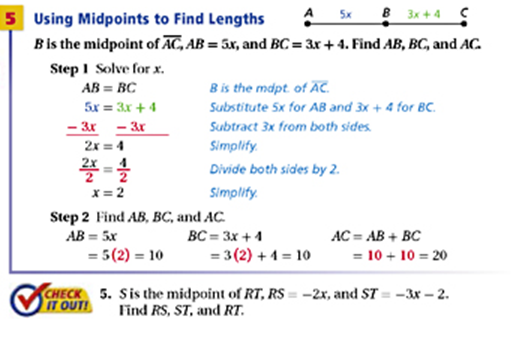
The picture of rectangle ABCD below shows all three rules listed above.

Sample:
In rectangle ABCD below, diagonals AC and BD intersect at point R. If AR = 2x - 6 and CR = x + 10, find BD.

Since the diagonals of a rectangle bisect each other, we can say that
AR = CR.
We equate the values of AR and CR and solve for x.
2x - 6 = x + 10
2x - x = 10 + 6
x = 16
Use either of the given equations to determine that each segment equals 26. Since they are all equal, BD = 26.

Rhombus

A rhombus is a parallelogram with 4 congruent or equal sides. A rhombus has the following rules:
(1) All the rules of a parallelogram.
(2) Four sides that have the same length.
(3) Diagonals that intersect at right angles.
(4) Diagonals that bisect opposite pairs of angles.

Sample:
Given that ABCD is a rhombus and the measure of angle D = 60 degrees.
Find the measure of angles A and B.

Solution:
Triangle ABC is isosceles since line segment AB is congruent to line segment BC. Then we can say that the base angles of triangle ABC must be congruent or equal. Since we know the diagonal bisects the angles A and C, we must have two congruent triangles here. If so, then the measure of angle D = the measure of angle B = 60 degrees.

In triangle AEB, angle AEB is a right triangle because the diagonals of a rhombus are perpendicular to each other. Since the sum of the degree measures of the angles of a triangle is 180 degrees, we can say that the measure of angle A must be 30 degrees. How do we get 30?

Measure of angle A = 180 - 90 degrees - 60 degrees
Measure of angle A = 30 degrees

Square

A square is a parallelogram with 4 right angles and 4 sides that have the same length. A square has all the rules of a rectangle and a rhombus as shown in square ABCD below.

Sample:
In square ABCD, AB = x + 4. What is the perimeter of square ABCD?
Solution:
A square has the same length on all 4 sides.
We can use the formula P = side times 4, or P = 4s, where P = perimeter and s = side of square.
P = 4s
P = 4(x + 4)....We apply the distributive rule here and get
P = 4x + 16
Our perimeter is 4x + 16.

Exterior Angles of Polygons

The Exterior Angle is the angle between any side of a shape,
and a line extended from the next side.

Note: when you add up the Interior Angle and Exterior Angle you get a straight line, 180°. (See Supplementary Angles)

Polygons

A Polygon is any flat shape with straight sides

The Exterior Angles of a Polygon add up to 360°
		In other words the exterior angles add up to one full revolution (Exercise: try this with a square, then with some interesting polygon you invent yourself.) Note: This rule only works for simple polygons

Here is another way to think about it: Each lines changes direction until you eventually get back to the start:

Interior Angles of Polygons

An Interior Angle is an angle inside a shape.

Triangles

The Interior Angles of a Triangle add up to 180°

90° + 60° + 30° = 180°	80° + 70° + 30° = 180°
It works for this triangle!	Let's tilt a line by 10° ... It still works, because one angle went up by 10°, but the other went down by 10°

Quadrilaterals (Squares, etc)

(A Quadrilateral has 4 straight sides)

90° + 90° + 90° + 90° = 360°	80° + 100° + 90° + 90° = 360°
A Square adds up to 360°	Let's tilt a line by 10° ... still adds up to 360°!
The Interior Angles of a Quadrilateral add up to 360°

Because there are Two Triangles in a Square

The interior angles in this triangle add up to 180° (90°+45°+45°=180°)

... and for this square they add up to 360° ... because the square can be made from two triangles!

Pentagon

		A pentagon has 5 sides, and can be made from three triangles, so you know what ... ... its interior angles add up to 3 × 180° = 540° And if it is a regular pentagon (all angles the same), then each angle is 540° / 5 = 108° (Exercise: make sure each triangle here adds up to 180°, and check that the pentagon's interior angles add up to 540°)
	The Interior Angles of a Pentagon add up to 540°

The General Rule

Each time we add a side (triangle to quadrilateral, quadrilateral to pentagon, etc), we add another 180° to the total:

			If it is a Regular Polygon (all sides are equal, all angles are equal)
Shape	Sides	Sum of Interior Angles	Shape	Each Angle
Triangle	3	180°		60°
Quadrilateral	4	360°		90°
Pentagon	5	540°		108°
Hexagon	6	720°		120°
Heptagon (or Septagon)	7	900°		128.57...°
Octagon	8	1080°		135°
Nonagon	9	1260°		140°
...	...	..	...	...
Any Polygon	n	(n-2) × 180°		(n-2) × 180° / n

So the general rule is:

Sum of Interior Angles = (n-2) × 180°

Each Angle (of a Regular Polygon) = (n-2) × 180° / n

Perhaps an example will help:

Example: What about a Regular Decagon (10 sides) ?

Sum of Interior Angles = (n-2) × 180°   = (10-2)×180° = 8×180° = 1440° And it is a Regular Decagon so: Each interior angle = 1440°/10 = 144°

Note: Interior Angles are sometimes called "Internal Angles"

Slope of the line

One of the most important properties of a straight line is in how it angles away from the horizontal. This concept is reflected in something called the "slope" of the line.
Let's take a look at the straight line y = ( ²/₃ ) x – 4. Its graph looks like this:

To find the slope, we will need two points from the line.
Pick two x's and solve for each corresponding y: If, say, x = 3, then y = ( ²/₃ )(3) – 4 = 2 – 4 = –2. If, say, x = 9, then y = ( ²/₃ )(9) – 4 = 6 – 4 = 2. (By the way, I picked the x-values to be multiples of three because of the fraction. It's not a rule that you have to do that, but it's a helpful technique.) So the two points (3, –2) and (9, 2) are on the line y = ( ²/₃ )x – 4.
To find the slope, you use the following formula:

(Why "m" for "slope", rather than, say, "s"? The official answer is: Nobody knows.)
The subscripts merely indicate that you have a "first" point (whose coordinates are subscripted with a "1") and a "second" point (whose coordinates are subscripted with a "2"); that is, the subscripts indicate nothing more than the fact that you have two points to work with. It is entirely up to you which point you label as "first" and which you label as "second". For computing slopes with the slope formula, the important thing is that you subtract the x's and y's in the same order. For our two points, if we choose (3, –2) to be the "first" point, then we get the following:

The first y-value above, the –2, was taken from the point (3, –2) ; the second y-value, the 2, came from the point (9, 2); the x-values 3 and 9 were taken from the two points in the same order. If we had taken the coordinates from the points in the opposite order, the result would have been exactly the same value:

As you can see, the order in which you list the points really doesn't matter, as long as you subtract the x-values in the same order as you subtracted the y-values. Because of this, the slope formula can be written as it is above, or alternatively it can be written as:

Copyright © Elizabeth Stapel 2000-2011 All Rights Reserved

Let me emphasize: it does not matter which of the two formulas you use or which point you pick to be "first" and which you pick to be "second". The only thing that matters is that you subtract your x-values in the same order as you had subtracted your y-values.

---

Technically, the equivalence of the two slope formulas above can be proved by noting that:

y₁ – y₂ = –y₂ + y₁ = –(y₂ – y₁)
x₁ – x₂ = –x₂ + x₁ = –(x₂ – x₁)

Doing the subtraction in the so-called "wrong" order serves only to create two "minus" signs which cancel out. The upshot: Don't worry too much about which point is the "first" point, because it really doesn't matter. (And please don't send me an e-mail claiming that the order does somehow matter, or that one of the above two formulas is somehow "wrong". If you think I'm wrong, plug pairs of points into both formulas, and try to prove me wrong! And keep on plugging until you "see" that the mathematics is in fact correct.)

---

Let's find the slope of another line equation:

• Find the slope of  y = –2x + 3.

Graphing the line, it looks like this:

I'll pick a couple of values for x, and find I'll find the corresponding values for y. Picking x = –1, I get y = –2(–1) + 3 = 2 + 3 = 5. Picking x = 2, I get y = –2(2) + 3 = –4 + 3 = –1. Then the points (–1, 5) and (2, –1) are on the line y = –2x + 3. The slope of the line is then calculated as:

Now YOU try it!

---

Scroll back up this page and look at those equations and their graphs. For the first equation, y
= ( ²/₃ )x – 4, the slope was m = ²/₃. And the line, as you moved from left to right along the x-axis, was heading up toward the top of the drawing; technically, the line was "increasing". For the second line, y = –2x + 3, the slope was m = –2. And the line, as you moved from left to right along the x-axis, was heading down toward the bottom of the drawing; technically, the line was "decreasing". This relationship is always true: Increasing lines have positive slopes, and decreasing lines have negative slopes. Always!
This fact can help you check your calculations: if you calculate a slope as being negative, but you can see from the graph that the line is increasing (so the slope must be positive), you know you need to re-do your calculations. Being aware of this connection can save you points on a test because it will enable you to check your work before you hand it in.

Increasing lines have positive slopes; decreasing lines have negative slopes. With this in mind, consider the following horizontal line: y = 4 Its graph is shown to the right.

Is the horizontal line going up; that is, is it an increasing line? No, so its slope won't be positive. Is the horizontal line going down; that is, is it a decreasing line? No, so its slope won't be negative. What number is neither positive nor negative? Zero! So the slope of this horizontal line is zero. Let's do the calculations to confirm this value. Using the points (–3, 4) and (5, 4), the slope is:

This relationship is true for every horizontal line: a slope of zero means the line is horizontal, and a horizontal line means you'll get a slope of zero. (By the way, all horizontal lines are of the form "y = some number", and the equation "y = some number" always graphs as a horizontal line.)

Now consider the vertical line x = 4: Is the vertical line going up on one end? Well, kind of. Is the vertical line going down on the other end? Well, kind of. Is there any number that is both positive and negative? Nope.

Verdict: vertical lines have NO SLOPE. In particular, the concept of slope simply does not work for vertical lines. The slope doesn't exist! Let's do the calculations. I'll use the points (4, 5) and (4, –3); the slope is:

(We can't divide by zero, which is of course why this slope value is "undefined".)
This relationship is always true: a vertical line will have no slope, and "the slope is undefined" means that the line is vertical. (By the way, all vertical lines are of the form "x = some number", and "x = some number" means the line is vertical. Any time your line involves an undefined slope, the line is vertical, and any time the line is vertical, you'll end up dividing by zero if you try to compute the slope.)
Warning: It is very common to confuse these two lines and their slopes, but they are very different. Just as "horizontal" is not at all the same as "vertical", so also "zero slope" is not at all the same as "no slope". The number "zero" exists, so horizontal lines do indeed have a slope. But vertical lines don't have any slope; "slope" just doesn't have any meaning for vertical lines. It is very common for tests to contain questions regarding horizontals and verticals. Don't mix them up!
Parallel lines and their slopes are easy. Since slope is a measure of the angle of a line from the horizontal, and since parallel lines must have the same angle, then parallel lines have the same slope — and lines with the same slope are parallel.

Perpendicular lines are a bit more complicated. If you visualize a line with positive slope (so it's an increasing line), then the perpendicular line must have negative slope (because it will be a decreasing line). So perpendicular slopes have opposite signs. The other "opposite" thing with perpendicular slopes is that their values are reciprocals; that is, you take the one slope value, and flip it upside down. Put this together with the sign change, and you get that the slope of the perpendicular line is the "negative reciprocal" of the slope of the original line — and two lines with slopes that are negative reciprocals of each other are perpendicular to each other. In numbers, if the one line's slope is m = ⁴/₅, then the perpendicular line's slope will be m = ^–5/₄. If the one line's slope is m = –2, then the perpendicular line's slope will be m = ¹/₂.
In your homework, you will probably be given some pairs of points, and be asked to state whether the lines through the pairs of points are "parallel, perpendicular, or neither". To answer the question, you'll have to calculate the slopes and compare them. Here's how that works:

• One line passes through the points (–1, –2) and (1, 2); another line passes through the points (–2, 0) and (0, 4). Are these lines parallel, perpendicular, or neither?

To answer this question, I'll find the slopes.

Since these two lines have identical slopes, then these lines are parallel.

• One line passes through the points (0, –4) and (–1, –7); another line passes through the points (3, 0) and (–3, 2). Are these lines parallel, perpendicular, or neither?

I'll find the values of the slopes. Copyright © Elizabeth Stapel 2000-2011 All Rights Reserved

 
If I were to flip the "3" and then change its sign, I would get " ^–1/₃". In other words, these slopes are negative reciprocals, so the lines through the points are perpendicular.

• One line passes through the points (–4, 2) and (0, 3); another line passes through the points (–3, –2) and (3, 2). Are these lines parallel, perpendicular, or neither?

I'll find the slopes.

 
These slope values are not the same, so the lines are not parallel. The slope values are not negative reciprocals either, so the lines are not perpendicular. Then the answer is "neither".

Warning: When asked a question of this type ("are they parallel or perpendicular?"), do not start drawing pictures. If the lines are close to being parallel or close to being perpendicular (or if you draw the lines messily), you can very-easily get the wrong answer from your picture. Besides, they're not asking if the lines look parallel or perpendicular; they're asking if the lines actually are parallel or perpendicular. To be sure of your answer, do the algebra.

Conditional statement

If-then statement

When we previously discussed inductive reasoning we based our reasoning on examples and on data from earlier events. If we instead use facts, rules and definitions then it's called deductive reasoning.
We will explain this by using an example.
If you get good grades then you will get into a good college.
The part after the "if": you get good grades - is called a hypotheses and the part after the "then" - you will get into a good college - is called a conclusion.
Hypotheses followed by a conclusion is called an If-then statement or a conditional statement.
This is noted as

This is read - if p then q.
A conditional statement is false if hypothesis is true and the conclusion is false. The example above would be false if it said "if you get good grades then you will not get into a good college".
If we re-arrange a conditional statement or change parts of it then we have what is called a related conditional.
Example
Our conditional statement is: if a population consists of 50% men then 50% of the population must be women.

If we exchange the position of the hypothesis and the conclusion we get a converse statement: if a population consists of 50% women then 50% of the population must be men.

If both statements are true or if both statements are false then the converse is true. A conditional and its converse do not mean the same thing
If we negate both the hypothesis and the conclusion we get a inverse statement: if a population do not consist of 50% men then the population do not consist of 50% women.

The inverse is not true juest because the conditional is true. The inverse always has the same truth value as the converse.
We could also negate a converse statement, this is called a contrapositive statement:  if a population do not consist of 50% women then the population do not consist of 50% men.

The contrapositive does always have the same truth value as the conditional. If the conditional is true then the contrapositive is true.
A pattern of reaoning is a true assumption if it always lead to a true conclusion. The most common patterns of reasoning are detachment and syllogism.
Example
If we turn of the water in the shower, then the water will stop pouring.
If we call the first part p and the second part q then we know that p results in q. This means that if p is true then q will also be true. This is called the law of detachment and is noted:

The law of syllogism tells us that if p → q and q → r then p → r is also true.
This is noted:

Example
If the following statements are true:
If we turn of the water (p), then the water will stop pouring (q). If the water stops pouring (q) then we don't get wet any more (r).
Then the law of syllogism tells us that if we turn of the water (p) then we don't get wet (r) must be true.

Razonamiento inductivo y conjeturas

Angles – measurement, construction, estimation

When two lines meet at a point, they make an angle. The two lines are called the arms of the angle and the point is called the vertex.

Detailed description

Two angles are created. One angle falls inside the arms, and the other angle falls outside them.

Detailed description

To measure the angle we see how much one of the lines must be turned through the shaded area to get to the other line.
An understanding of angles forms the basis of much of the geometry you will meet in upper primary and in secondary school. Geometry, including angles, has been used throughout history. The Egyptians must have had a very good knowledge of angles to construct the pyramids so perfectly.

Measuring and Constructing Segments

Definitions:

Distance - The distance between any two points is the absolute value of the difference of the coordinates.
Length - The distance between two points.
Congruent segments - Segments that have the same length.
Coordinates - A point on a grid or graph.
Construction - A way of creating a figure that is more precise.
Midpoint - The point that bisects or divides the segment into two congruent segments.

Examples